LeetCode: 547. 省份数量

友情支持

如果您觉得这个笔记对您有所帮助，看在D瓜哥码这么多字的辛苦上，请友情支持一下，D瓜哥感激不尽，😜

支付宝

有些打赏的朋友希望可以加个好友，欢迎关注D 瓜哥的微信公众号，这样就可以通过公众号的回复直接给我发信息。

wx jikerizhi

公众号的微信号是: jikerizhi。因为众所周知的原因，有时图片加载不出来。如果图片加载不出来可以直接通过搜索微信号来查找我的公众号。

547. 省份数量

LeetCode - 547. 省份数量

有 n 个城市，其中一些彼此相连，另一些没有相连。如果城市 a 与城市 b 直接相连，且城市 b 与城市 c 直接相连，那么城市 a 与城市 c 间接相连。

省份是一组直接或间接相连的城市，组内不含其他没有相连的城市。

给你一个 n x n 的矩阵 isConnected ，其中 isConnected[i][j] = 1 表示第 i 个城市和第 j 个城市直接相连，而 isConnected[i][j] = 0 表示二者不直接相连。

返回矩阵中省份的数量。

示例 1：

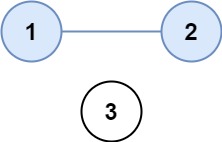
0547 01

输入：isConnected = [[1,1,0],[1,1,0],[0,0,1]]
输出：2

示例 2：

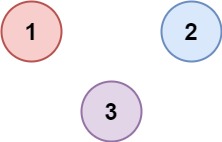
0547 02

输入：isConnected = [[1,0,0],[0,1,0],[0,0,1]]
输出：3

提示：

1 <= n <= 200
n == isConnected.length
n == isConnected[i].length
isConnected[i][j] 为 1 或 0
isConnected[i][i] == 1
isConnected[i][j] == isConnected[j][i]

思路分析

这就是一道典型的并查集题目，所谓的“返回省份数量”就是求解连通分量。这道题的连通性是通过一个矩阵表示的，所以，首先需要将这个矩阵转换成一个 Union Find Set 并查集中讲解的数组。由于 (i, j) 和 (j, i) 表示的含义一样，所以只需要扫描矩阵的右上部分或者左下部分即可。代码如下：

一刷

 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
/**
 * @author D瓜哥 · https://www.diguage.com
 * @since 2025-04-03 22:15:52
 */
public int findCircleNum(int[][] isConnected) {
  int len = isConnected.length;
  UnionFind un = new UnionFind(len);
  for (int i = 0; i < len; i++) {
    // 只处理矩阵右上半部分
    for (int j = len - 1; j > i; j--) {
      if (isConnected[i][j] == 1) {
        un.union(i, j);
      }
    }
  }
  return un.count();
}

private static class UnionFind {
  /**
   * 连通分量
   */
  int size;
  /**
   * 每个节点及对应的父节点
   */
  int[] parent;

  public UnionFind(int size) {
    this.size = size;
    parent = new int[size];
    for (int i = 0; i < size; i++) {
      parent[i] = i;
    }
  }

  /**
   * 连通分量
   */
  public int count() {
    return size;
  }

  /**
   * a 和 b 建立连接
   */
  public void union(int a, int b) {
    int ap = find(a);
    int bp = find(b);
    if (ap == bp) {
      return;
    }
    parent[ap] = bp;
    size--;
  }

  /**
   * 查找节点 a 的根节点
   */
  private int find(int a) {
    int ap = parent[a];
    if (ap != a) {
      List<Integer> path = new ArrayList<>();
      path.add(a);
      // 向上查找根节点
      while (ap != parent[ap]) {
        path.add(ap);
        ap = parent[ap];
      }
      // 路径压缩
      // 只有一步，无需缩短路径
      if (path.size() == 1) {
        return ap;
      }
      for (Integer idx : path) {
        parent[idx] = ap;
      }
    }
    return ap;
  }
}

LeetCode: 547. 省份数量

友情支持

547. 省份数量

思路分析

参考资料